Trigonometry All Formulas List
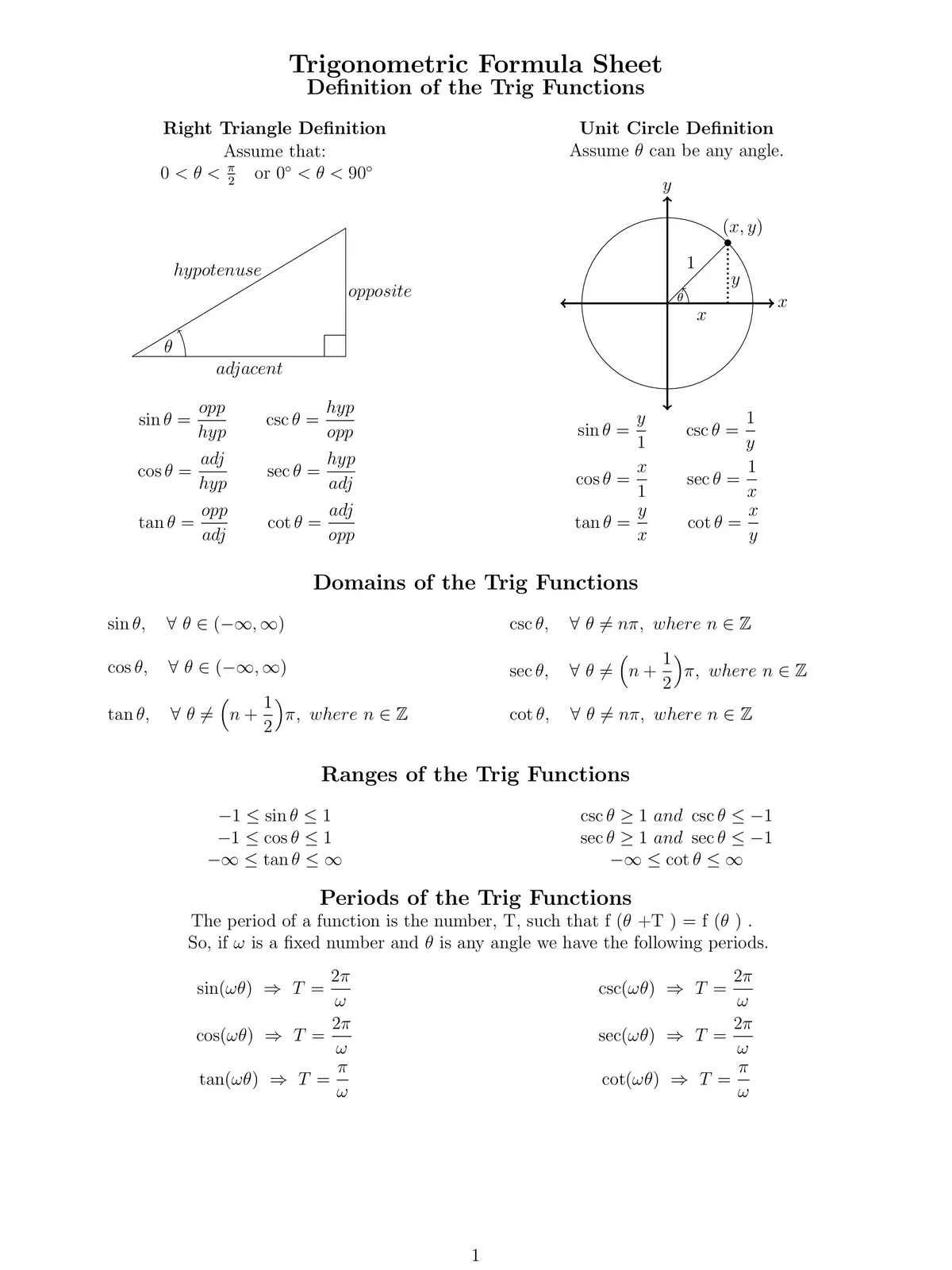
Trigonometry is a vital branch of mathematics that focuses on functions of angles and their application in calculations. In trigonometry, there are six main functions associated with angles: sine (sin), cosine (cos), tangent (tan), cotangent (cot), secant (sec), and cosecant (csc). These trigonometric functions are essential, especially when working with right triangles, and can be visualized as shown in the figure.
Understanding Trigonometry
The development of trigonometry arose from the need to calculate angles and distances in various fields, including astronomy, mapmaking, surveying, and artillery range-finding. Problems related to angles and distances in a single plane are studied in plane trigonometry. Meanwhile, when dealing with similar problems in three-dimensional space, we employ spherical trigonometry.
Trigonometry All Formulas List
Basic Trigonometry Formulas
- sin θ = Opposite Side / Hypotenuse
- cos θ = Adjacent Side / Hypotenuse
- tan θ = Opposite Side / Adjacent Side
- sec θ = Hypotenuse / Adjacent Side
- cosec θ = Hypotenuse / Opposite Side
- cot θ = Adjacent Side / Opposite Side
Periodic Identity of Trigonometric Angles
- sin(π/2 – A) = cos A & cos(π/2 – A) = sin A
- sin(π/2 + A) = cos A & cos(π/2 + A) = -sin A
- sin(3π/2 – A) = -cos A & cos(3π/2 – A) = -sin A
- sin(3π/2 + A) = -cos A & cos(3π/2 + A) = sin A
- sin(π – A) = sin A & cos(π – A) = -cos A
- sin(π + A) = -sin A & cos(π + A) = -cos A
- sin(2π – A) = -sin A & cos(2π – A) = cos A
- sin(2π + A) = sin A & cos(2π + A) = cos A
Cofunction Identity
- sin(90° – x) = cos x
- cos(90° – x) = sin x
- tan(90° – x) = cot x
- cot(90° – x) = tan x
- sec(90° – x) = cosec x
- cosec(90° – x) = sec x
Sum and Difference Trigonometric Formula
- sin(x + y) = sin(x) cos(y) + cos(x) sin(y)
- cos(x + y) = cos(x) cos(y) – sin(x) sin(y)
- tan(x + y) = (tan x + tan y) / (1 – tan x tan y)
- sin(x – y) = sin(x) cos(y) – cos(x) sin(y)
- cos(x – y) = cos(x) cos(y) + sin(x) sin(y)
- tan(x – y) = (tan x – tan y) / (1 + tan x tan y)
Double Angle Formula
- sin(2x) = 2 sin(x) cos(x) = 2 tan(x) / (1 + tan²(x))
- cos(2x) = cos²(x) – sin²(x) = (1 – tan²(x))(1 + tan²(x))
- cos(2x) = 2 cos²(x) – 1 = 1 – 2 sin²(x)
- tan(2x) = 2 tan(x) / (1 – tan²(x))
- sec(2x) = sec²(x) / (2 – sec²(x))
- csc(2x) = (sec(x) csc(x))²
Inverse Trigonometric Function
- sin⁻¹(−x) = −sin⁻¹(x)
- cos⁻¹(−x) = π − cos⁻¹(x)
- tan⁻¹(−x) = −tan⁻¹(x)
- cosec⁻¹(−x) = −cosec⁻¹(x)
- sec⁻¹(−x) = π − sec⁻¹(x)
- cot⁻¹(−x) = π − cot⁻¹(x)
You can download all Trigonometry Maths Formulas List PDF using the link given below.